Предмет: Математика,
автор: sevcenkovaleria081
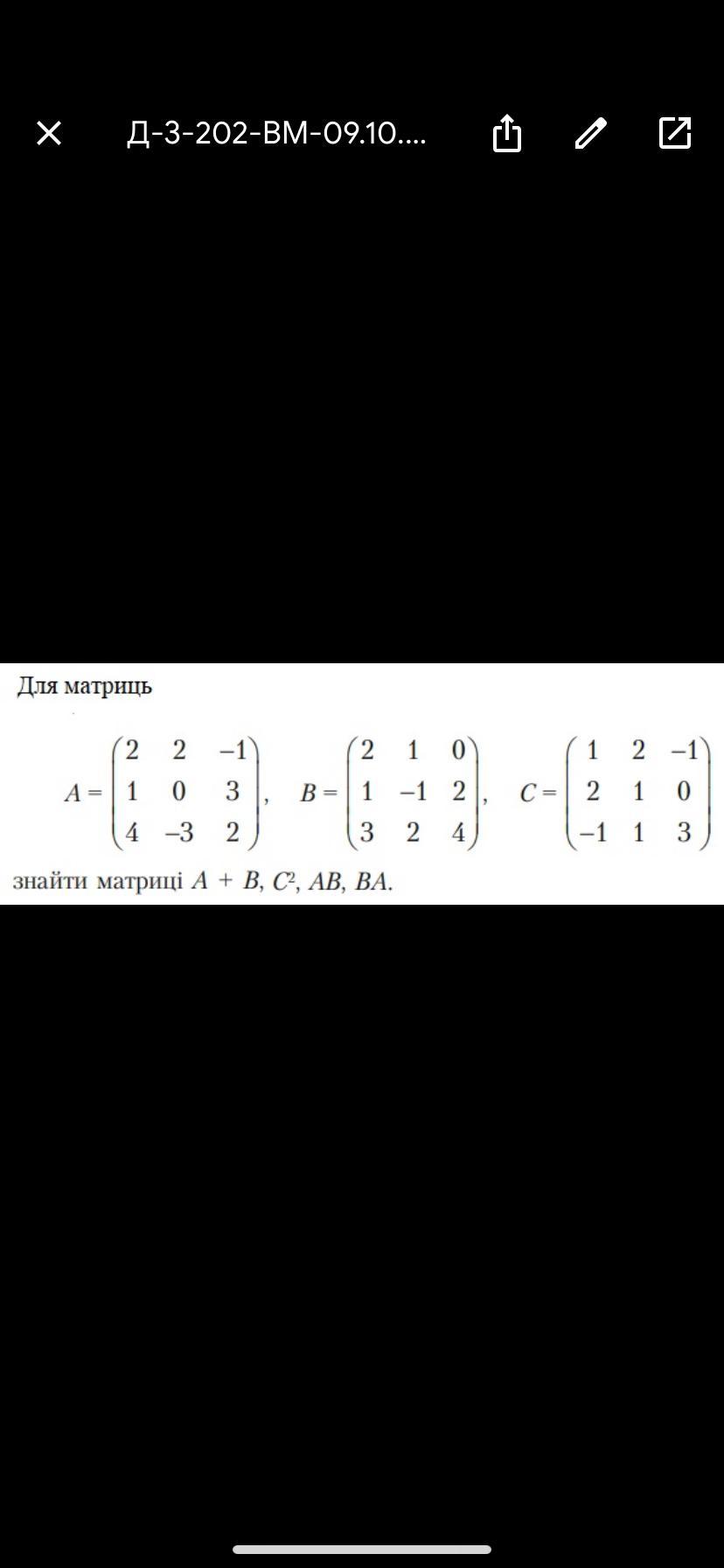
Знайти матриці, ДОПОМОЖІТЬ БУДЬ ЛАСКА
Приложения:
Ответы
Автор ответа:
0
Ответ:
.......
himikomat:
надеюсь помог
Похожие вопросы
Предмет: Литература,
автор: diniskovernik
Предмет: Українська мова,
автор: butd48498
Предмет: Химия,
автор: barseghyan17829
Предмет: Английский язык,
автор: Аноним
Предмет: Русский язык,
автор: aminanusip