Предмет: Математика,
автор: kalisanjar325
[11x + y = 5
[3x-2y = 40
помогите пожалуйста дам 15 балов
Ответы
Автор ответа:
1
Ответ:
We can use the method of substitution to solve for x and y. From the first equation, we can solve for y:
y = 5 -11x
Then we can substitute this expression for y in the second equation:
3x - 2(5 - 11x) = 40
Simplifying:
3x - 10 + 22x = 40
2511x
Now we can substitute this expression for y in the second equation:
3x - 2(5 - 11x) = 40
Simplifying this equation, we get:
3x - 10 + 22x = 40
25x = 50
x = 2
Now we can substitute this value for x in the first equation to solve for y:
11(2) + y = 5
y = -17
Therefore, the solution to the system of equations is x = 2 and y = -17.
Пошаговое объяснение:
сори что на английском мне так удобно
Автор ответа:
0
Ответ:
(2;-18) вот это ответ на уравнение
Приложения:
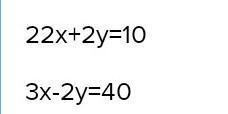

Похожие вопросы
Предмет: Математика,
автор: galymkazrahym
Предмет: Українська мова,
автор: sasakirsenin
Предмет: Математика,
автор: aksaodat3
Предмет: Математика,
автор: michaylo2005
Предмет: Українська література,
автор: ThePoopAuf