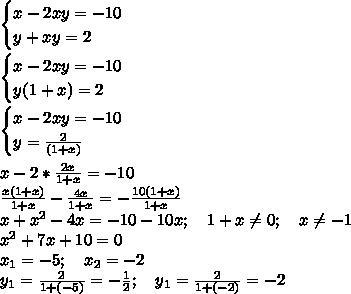(xy=2(x+y) Lorry10x+y-27=10y+x
Ответы
Ответ:
(x, y) = (2 - 2√3, 4 - 2√3) or (2 + 2√3, 4 + 2√3)
Объяснение:
Quadratic Solution for Two Equations
nealglie_41
(xy=2(x+y) Lorry10x+y-27=10y+x
To solve the given equations:
xy = 2(x+y) --------(1)
Lorry10x+y-27=10y+x --------(2)
Let's simplify equation (2) by rearranging the terms:
10x - x + y - 10y = 27
9x - 9y = 27
x - y = 3 --------(3)
Now, we can solve for x and y by substituting equation (3) into equation (1):
y(x-y) = 2(x+y)
xy - y^2 = 2x + 2y
Substituting x - y = 3, we get:
xy - y^2 = 2(x+y)
xy - y^2 = 2(3+y)
xy - y^2 = 6 + 2y
Rearranging, we get a quadratic equation:
y^2 - (2+x)y + 6 = 0
Using the quadratic formula, we get:
y = (2+x) ± √(x^2 - 4x - 8) / 2
For y to be a real number, the discriminant (x^2 - 4x - 8) must be non-negative:
x^2 - 4x - 8 ≥ 0
Solving for x, we get:
x ≤ 2 - 2√3 or x ≥ 2 + 2√3
Substituting these values of x into the quadratic formula, we get corresponding values of y. Thus, the solution to the system of equations is:
(x, y) = (2 - 2√3, 4 - 2√3) or (2 + 2√3, 4 + 2√3)
Ответ:
Объяснение:
даш каронку