Предмет: Математика,
автор: agunda12
Доказать справедливость равенства
Приложения:
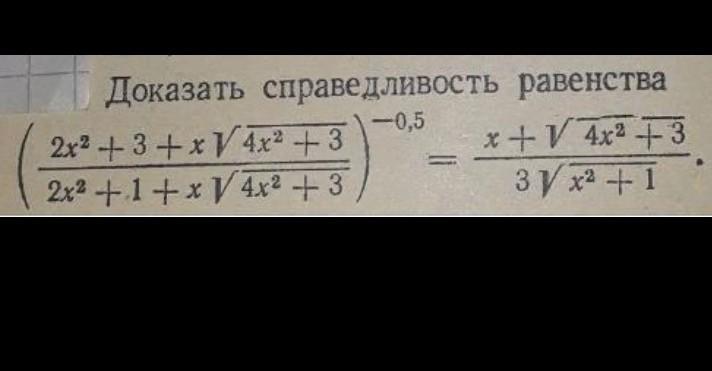
Ответы
Автор ответа:
2
Похожие вопросы
Предмет: Английский язык,
автор: Дpaтути
Предмет: Русский язык,
автор: artempasura
Предмет: Другие предметы,
автор: ladytchizhova
Предмет: Математика,
автор: dimacelujkin
Предмет: Русский язык,
автор: vivtash2005