Предмет: Алгебра,
автор: esstry
100 БАЛЛОВ. СРОЧНО. Найти 7 производных. С решением, пожалуйста.
Приложения:
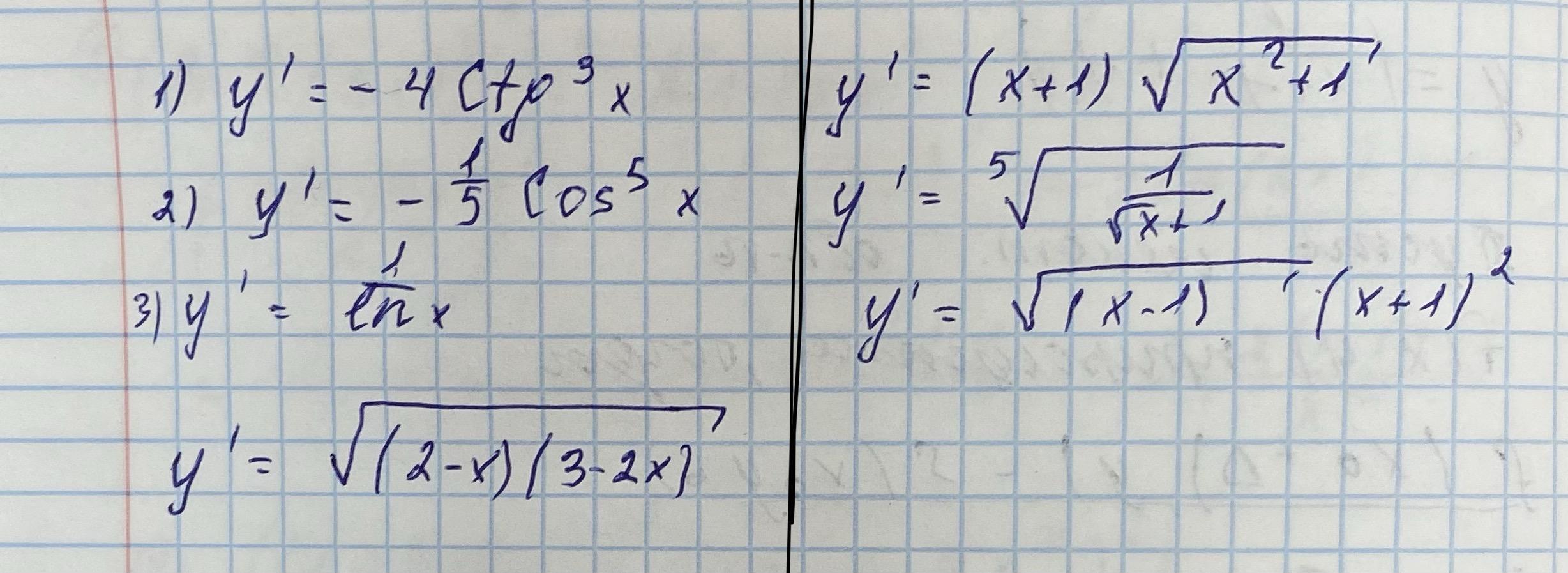
Ответы
Автор ответа:
1
Ответ:
Похожие вопросы
Предмет: Қазақ тiлi,
автор: галым1
Предмет: Русский язык,
автор: ArsFeed
Предмет: Окружающий мир,
автор: тинур
Предмет: Русский язык,
автор: sheraliberdiev
Предмет: Информатика,
автор: Бенджамин11