Предмет: Алгебра,
автор: yulmel123
Допомжіть, помогитееееее, пжжж
Приложения:
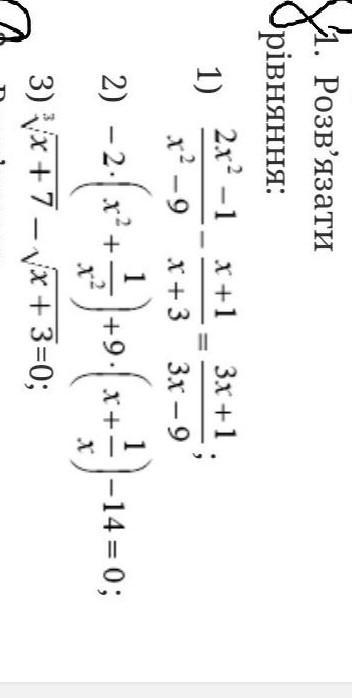
Ответы
Автор ответа:
1
Ответ:
Похожие вопросы
Предмет: Русский язык,
автор: Ramses321
Предмет: Технология,
автор: евгешка61
Предмет: Русский язык,
автор: irinalichacheva
Предмет: Математика,
автор: swatcamgg