Предмет: Алгебра,
автор: alex111207shadski
1 вариант пж очень надо
Приложения:
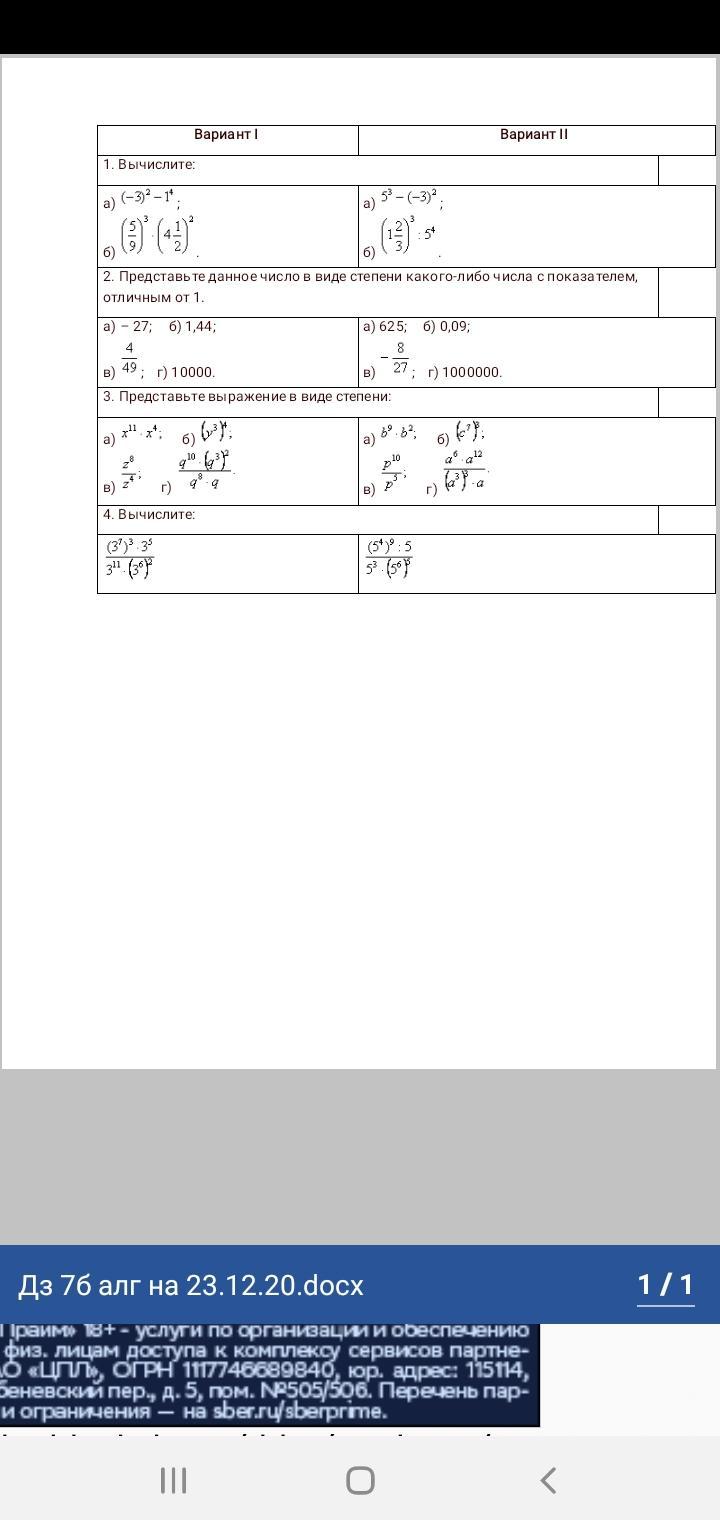
Ответы
Автор ответа:
1
1.
а)
б)
2.
а)
б)
в)
г)
3.
а)
б)
в)
г)
4)
Похожие вопросы
Предмет: Математика,
автор: Аноним
Предмет: Другие предметы,
автор: UsEnOk
Предмет: Русский язык,
автор: ponybrony04
Предмет: Математика,
автор: fedia5
Предмет: Математика,
автор: Данил4532