Даю 52 балла
Найти производные функций
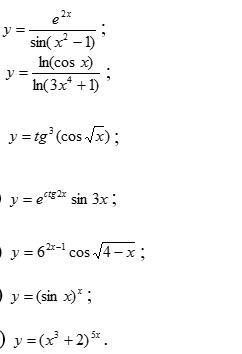
Ответы
Ответ:
1)
y=e^2x/sin(x^2-1) =
y' = d/dx(e^2x/sin(x^2-1)) =
y' = d/dx(e^2x)*sin(x^2-1)-e^2x*d/dx(sin(x^2-1))/sin(x^2-1)^2 =
y' = e^2x*2sin(x^2-1)-e^2x*cos(x^2-1)*2x/sin(x^2-1)^2 (упрощаем) =
y' = 2e^2x*sin(x^2-1)-2xe^2x*cos(x^2-1)/sin(x^2-1)^2.
2)
я сразу к решению ( не буду переписывать сам пример)
y' = d/dx(ln(cos(x))/ln(3x^4+1)) =
y' = d/dx(ln(cos(x)))*ln(3x^4+1)-ln(cos(x))*d/dx(ln(3x^4+1))/ln(3x^4+1)^2 =
y' = 1/cos(x)*(-sin(x))*ln(3x^4+1)-ln(cos(x))*1/3x^4+1 3*4x^3/ln(3x^4+1)^2 =
y' = - (3x^4+1)*sin(x)ln(3x^4+1)+12ln(cos(x))*x^3*cos(x)/(3x^4+1)*cos(x)ln(3x^4+1)^2
3)
y' = d/dx(tg(cos(корень x))^3) =
y' = d/dg(g^3)*d/dx(tg(cos(корень x))) =
y' = 3g^2*sec(cos(корень x))^2*(-sin(корень x)*1/2корень x) =
y' = 3tg(cos(корень x))^2sec(cos(корень x))^2*(-sin(корень x)*1/2корень x) =
y' = - 3/sin(cos(корень x))^2sin(корень x)/2корень x cos(cos(корень x))^4
4)
y ' = d/dx(e^ctg(2x)*sin(3x)) =
y' = d/dx(e^ctg(2x))*sin93x)+e^ctg(2x)+d/dx9sin(3x)) =
y' = e^ctg(2x)*(-csc(2x)^2*2)*sin(3x)+e^ctg(2x)*cos(3x)*3 =
y' = -2e^ctg(2x)*csc(2x)^2sin(3x)+3e^ctg(2x)*cos(3x)
5)
y' = d/dx(6^2x-1*cos(корень 4-x)) =
y' = d/dx(6^2x-1)*cos(корень 4-x)+6^2x-1*d/dx(cos(корень 4-x)) =
y' = ln(6)*6^2x-1*2cos(корень 4-x)+6^2x+1*(-sin(корень 4-x)*1/2 корень 4-x *(-1)) =
y' = 2ln(6)*6^2x-1*cos(корень 4-x)+6^2x-1*sin(корень 4-x)/2 корень 4-x
6)
y' = d/dx(sin(x)^x) =
y' = d/dx((e^ln9sin(x)))^x) =
y' = d/dg(e^ln9sin(x))*x) =
y' = e^g*(1/sin(x) *cos9x)*x+ln(sin(x))) =
y' = e^ln(sin(x))*x*(1/sin(x) *cos(x)*x+ln(sin(x))) =
y' = sin(x)^x-1cos(x)*x+sin(x)^xkn(sin(x))
7)
y' = d/dx((x^3+2)^5x) =
y' = d/dx((e^ln(x^3+2))^5x) =
y' = d/dx(e^ln(x^3+2) *5x) =
y' = d/dg(e^g)*d/dx(ln(x^3+2)*5x) =
y' = e^g*(1/x^3+2 * 3x^2*5x+ln(z^3+2)*5) =
y' = e^ln(x^3+2)*5x *(1/x^3+2 *3x^2*5x+ln(3x^2+2)*5) =
y' = (x^3+2)^5x *(15x^3+5ln(x^3+2)*(x^3+2))/x^3+2