Предмет: Алгебра,
автор: minovaksyushashai
НАЙТИ ПРОИЗВОДНУЮ ФУНКЦИИ
Приложения:
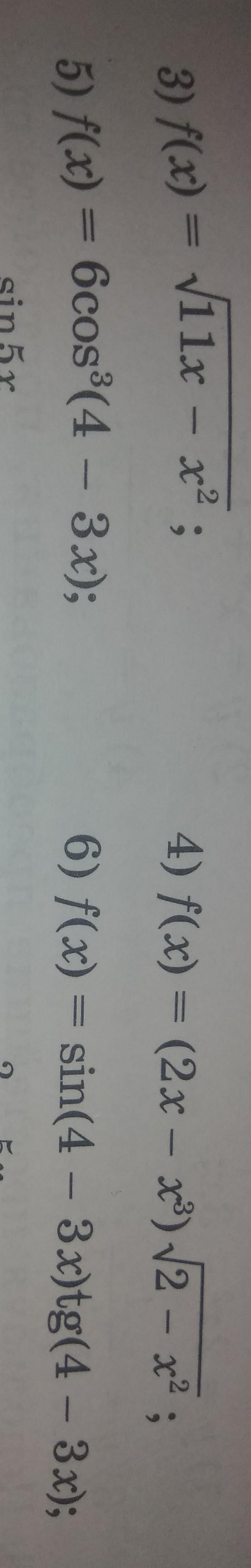
Ответы
Автор ответа:
3
Объяснение:
Похожие вопросы
Предмет: Другие предметы,
автор: devochka390
Предмет: ОБЖ,
автор: Аноним
Предмет: Математика,
автор: kaluzkanazar11
Предмет: Математика,
автор: sofiaIee2007031