Предмет: Алгебра,
автор: zvezdapezda10
Помогите решить систему уравнений
Приложения:
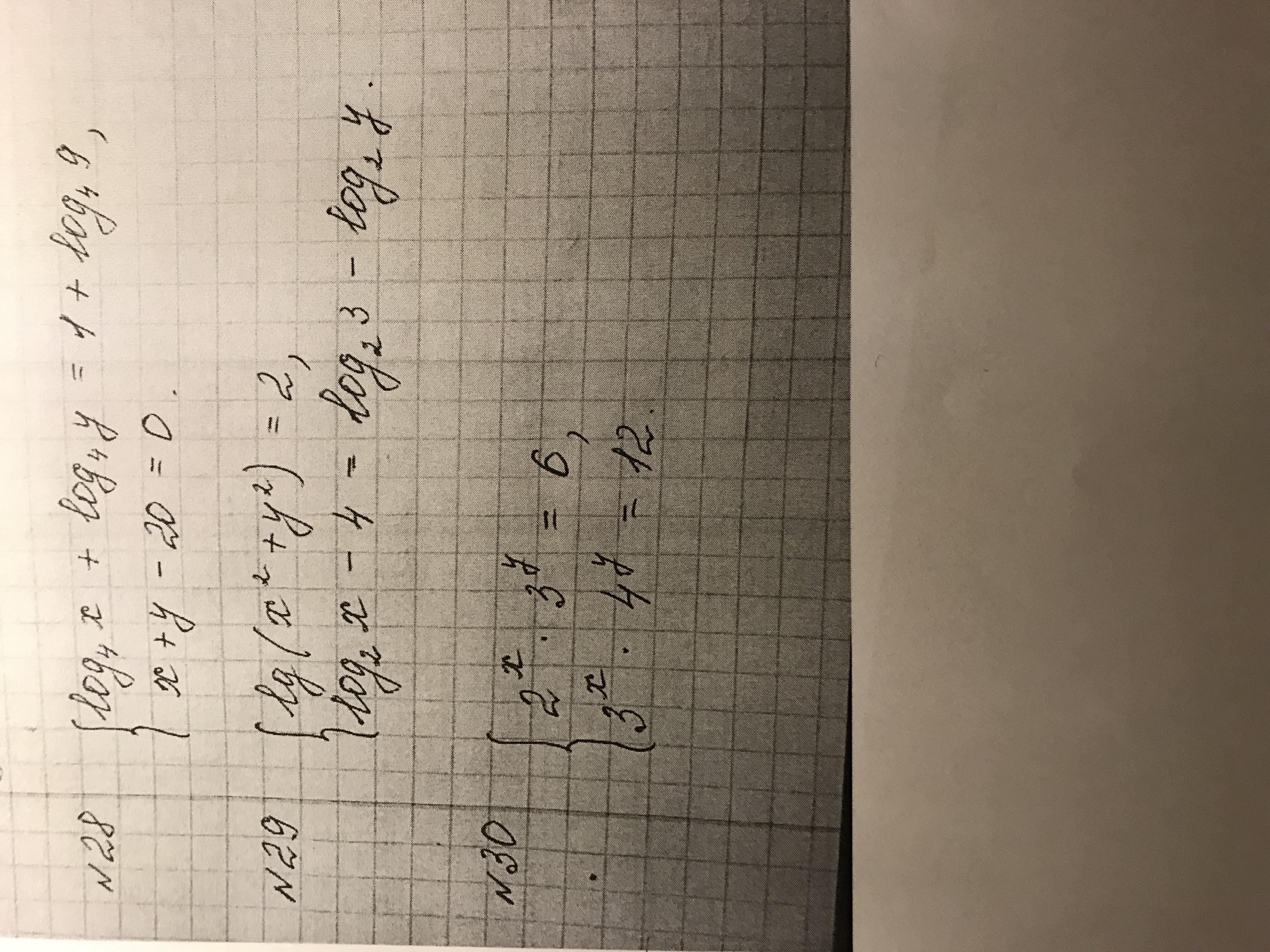
Ответы
Автор ответа:
2
Ответ:
Ответ:
Ответ:
Похожие вопросы
Предмет: Химия,
автор: v4dssh67vq
Предмет: Алгебра,
автор: model19055091
Предмет: Геометрия,
автор: Аноним
Предмет: Математика,
автор: лидия42