Предмет: Алгебра,
автор: Эльтит
Помогите решить 2 и 3 задание ! Подробно с пояснениями * желательно на бумаге*
Приложения:
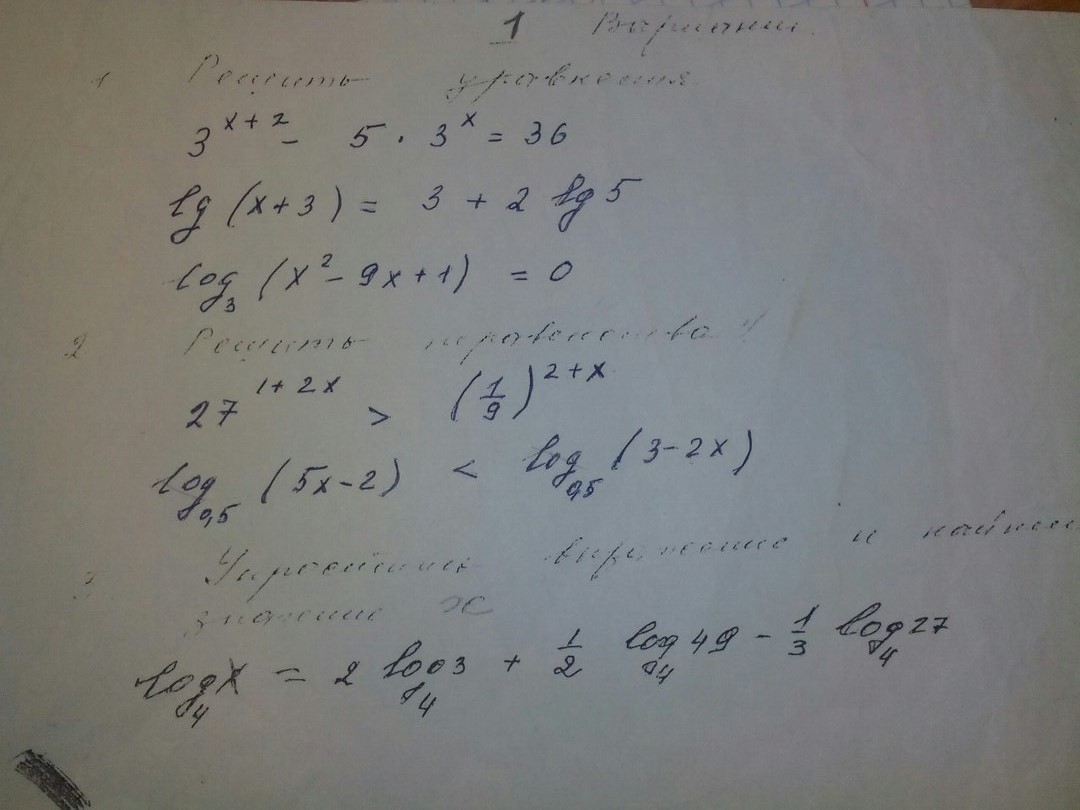
Ответы
Автор ответа:
0
Похожие вопросы
Предмет: Английский язык,
автор: anutasakalanskaa
Предмет: Английский язык,
автор: polisucsona16
Предмет: Английский язык,
автор: anutasakalanskaa
Предмет: Математика,
автор: vikalike