Предмет: Алгебра,
автор: nadtgh
ПОМОГИТЕ РЕШИТЬ НЕРАВЕНСТВО!!
Приложения:
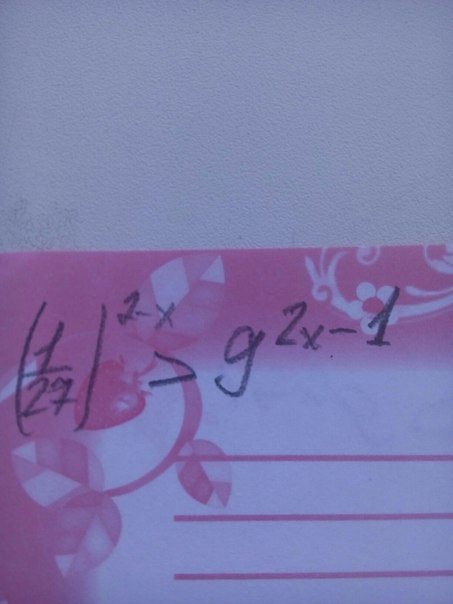
Ответы
Автор ответа:
1
NNNLLL54:
2(2-x)=4-2x ....
По условию 2(2х-1)=4х-2
Автор ответа:
1
Похожие вопросы
Предмет: Английский язык,
автор: АмиНКа1999
Предмет: Английский язык,
автор: VladiVit
Предмет: Другие предметы,
автор: Sergeyaaaa
Предмет: Английский язык,
автор: Аноним
Предмет: Литература,
автор: eroptank