Предмет: Алгебра, автор: ebri

Решите пожалуйста очень нужно

Приложения:

Ответы

Автор ответа: agelena

0

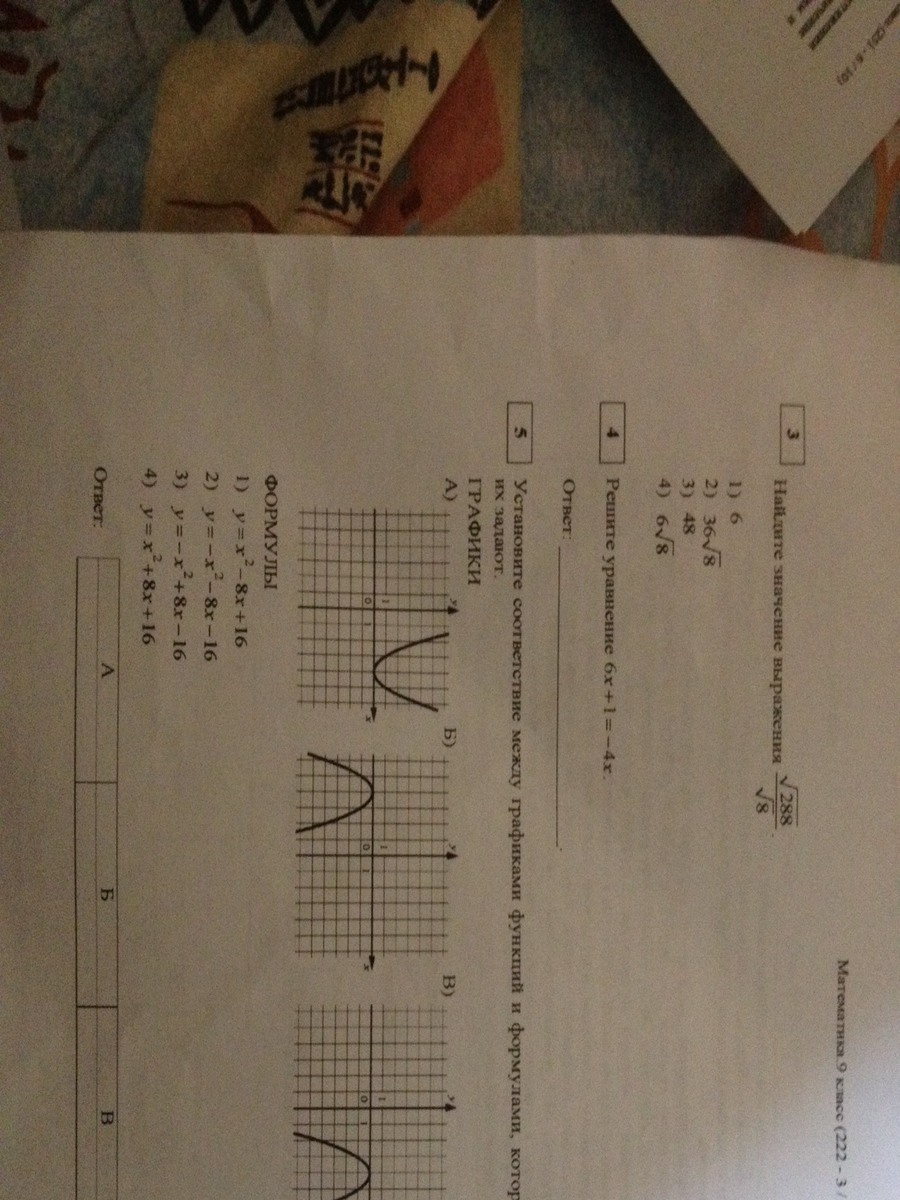
3.1)6
4.6x+1=-4x
6x+4x+1=0
10x=-1
x=-(1÷10)

Предыдущий вопрос

Следующий вопрос

Похожие вопросы

Предмет: Английский язык, автор: mg1998

!!!!!! переклад текст на українську мову
Newton's analysis of motion is summarized in his famous "three laws of motion".
In his great work, the Principia (published in 1687), Newton readily acknowledged
his debt to Galileo. In fact, Newton's first law of motion is very close to Galileo's
conclusions. It states that:
Every body continues in its state of rest or of uniform speed in a straight
line unless acted on by a nonzero net force.
The tendency of a body to maintain its state of rest or of uniform motion in a
straight line is called inertia. As a result, Newton's first law is often called the law
of inertia.
Newton's first law does not hold in every reference frame. For example, if your
reference frame is fixed in an accelerating car, an object such as a cup resting on the
dashboard may begin to move toward you (it stayed at rest as long as the car's
velocity remained constant). The cup accelerated toward you but neither you nor
anything else exerted a force on it in that direction. In such an accelerating
reference frame, Newton's first law does not hold. Reference frames in which
Newton's first law does hold are called inertial reference frames (the law of inertia is
valid in them). For most purposes, we can usually assume that reference frames fixed
on Earth's rotation, but usually it is close enough.) Any reference frame that moves
with constant velocity (say, a car or an airplane) relative to an inertial frame is also
an inertial reference frame. Reference frames where the law of inertia does not
hold, such as the accelerating reference frame discussed above, are called
noninertial reference frames. How can we be sure a reference frame is inertial or not?
By checking to see if Newton's first law holds.

1 год назад

Предмет: Окружающий мир, автор: katry1988

Как понять подготовить сообщение о представителе царство животных?

1 год назад

Предмет: Українська мова, автор: Stoianova1988

Помогитe составить слова из этих букв на украинском языке

1 год назад

Предмет: Литература, автор: Nelogi4niy

Какую первую фразу произнёс маленький принц при своём появлении?
"Маленький принц"
Пожалуйста.

7 лет назад

Предмет: Русский язык, автор: ematinaalisa

Помогите с русским
ворота тяжело закачались, раздался ужасающий скрип и треск, и левая воротина медленно отворилась. разбор 4
(повест., невоскл., грам.основа-

7 лет назад